Example of an Ethanol Dilution
"You need to prepare 500 ml of 40 % Ethanol from Ethanol (96 per cent) BP. What volume of Ethanol (96 per cent) BP do you need?
The long approach uses the definition of percent volume in volume:
"Percent Volume in Volume gives the number of millilitres
of a component in 100 millilitres of the total system."
"You need to prepare 500 ml of 40 % Ethanol from Ethanol (96 per cent) BP. What volume of Ethanol (96 per cent) BP do you need?
of a component in 100 millilitres of the total system.
of 40 % v/v Ethanol.
100 ml of the solution contains 40 ml of Absolute Ethanol. Thus:
500 ml of the solution contains 500/100 x 40 ml = 200 ml of Absolute Ethanol.
96.3 ml of Ethanol in every 100 ml of solution. Thus:
200 ml of Ethanol are in 200/96.3 x 100 ml
200 ml of Ethanol are in 207.7 ml of solution.
207.7 ml of Ethanol (96 per cent) BP are needed.
207.7 ml Ethanol (96 per cent) BP to 500 ml Water
The short approach uses the following equation:
Concentration1 x Quantity1 = Concentration2 x Quantity2
| Concentration1 | = Concentration of Original Solution |
| Quantity1 | = Quantity (mass or volume) of Original Solution |
| Concentration2 | = Concentration of New Solution |
| Quantity2 | = Quantity (mass of volume) of New Solution |
Click on Equation to Find Out Where It Comes From
Derivation of the Equation
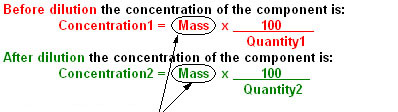
One definition of concentration expressed as a percent weight in volume is:
"Percent weight in volume is the mass - in grams - of a
component present in 100 millilitres of the total system"
This definition leads to the following equation:
Volume (in ml)
you haven't added anymore of the component.
Rearranging the Equations
Before Dilution
Quantity1
After Dilution
Quantity2
"You need to prepare 500 ml of 40 % Ethanol from Ethanol (96 per cent) BP. What volume of Ethanol (96 per cent) BP do you need?
Derivation of the Equation
One definition of concentration expressed as a percent weight in volume is:
"Percent weight in volume is the mass - in grams - of a
component present in 100 millilitres of the total system"
This definition leads to the following equation:
Volume (in ml)

you haven't added anymore of the component.
Rearranging the Equations
Before Dilution
Quantity1
After Dilution
Quantity2


You can solve this problem in two ways. The first approach is long and uses the definition of percent volume in volume. The second approach is shorter and uses a simple equation.